functions can be approximated by series
Comprehensive notes on Taylor Series 테일러 급수 포괄적 자료
University of Washington Math 126 자료
http://www.math.washington.edu/~m126/TaylorNotes.pdf
Taylor series is a representation of a function as an infinite sum of terms that are calculated from the values of the function's derivatives at a single point.
- Taylor series (물리과학)
- 임의의 함수를 점 x= a 부근에서 다항식으로 근사하는 방법.
- Taylor series (수리과학)
- 특정 함수식을 다항식으로 표현하는 방법.
"As the degree of the Taylor polynomial rises, it approaches the correct function. This image shows sin x (in black) and Taylor approximations, polynomials of degree 1, 3, 5, 7, 9, 11 and 13."
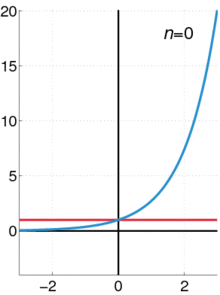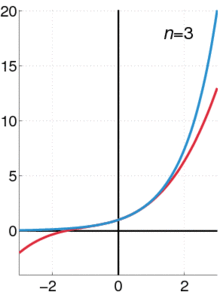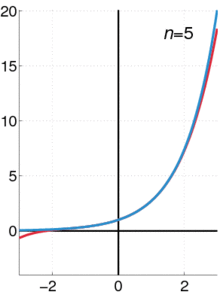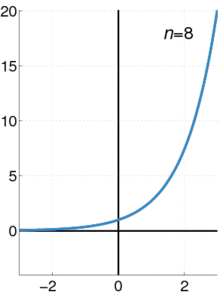
"The exponential function (in blue), and the sum of the first n+1 terms of its Taylor series at 0 (in red)."
Definition
The Taylor series of a real or complex function ƒ(x) that is infinitely differentiable in a neighborhood of a real or complex number a is the power series
which can be written in the more compact sigma notation as
where n! denotes the factorial of n and ƒ (n)(a) denotes the nth derivative of ƒ evaluated at the point a. The zeroth derivative of ƒ is defined to be ƒ itself and (x − a)0 and 0! are both defined to be 1. In the case that a = 0, the series is also called a Maclaurin series.
Fundamentals of Physics (PHYS 200) 16. The Taylor Series and Other Mathematical Concepts
무표정으로 재미있는 교수 my 이상형 쿸 ah 공부가 필요하다
http://www.youtube.com/watch?v=KzrdZD4EPXY
http://en.wikipedia.org/wiki/Taylor_series
'자연 과학' 카테고리의 다른 글
| higgs boson 힉스 보존 입자 존재하는가, 증명은 시간문제인가 (0) | 2011.12.16 |
|---|---|
| quark 분수의 전하를 가진 입자 쿼크 (0) | 2011.12.16 |
| metabolism 물질대사 (생화학) (0) | 2011.11.24 |
| Game theory 게임이론 (0) | 2011.11.23 |
| newtonian, non-newtonian fluid 뉴턴 유체, 비뉴턴 유체 - 실질적 활용 (0) | 2011.11.05 |




